11. Mass and Center of Mass

d. Electric Charge

1. Bar with Non-Uniform Charge Density

On the previous pages, we considered a bar with a non-uniform distribution of mass specified by a linear mass density \(\delta(x)\). We now consider a bar with a non-uniform distribution of electric charge specified by a linear electric charge density \(\delta_e(x)\). The main difference is that the mass density is always positive while the charge density can be positive or negative indicating that some parts of the bar have positive charge and other parts have negative charge.

The plot shows a bar from x equals a to b, with uneven density
which alternates between two colors which represent positive and negative
charge, showing the alternating electric charge density.

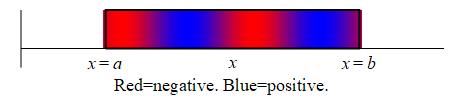
The computation of the total charge on the bar is exactly the same as the computation of the total mass but using \(\delta_e(x)\) instead of \(\delta(x)\). However, the total charge is usually called the net charge because the positive charge cancels with the negative charge leaving a net charge.

The net electric charge on a bar with linear charge density \(\delta_e(x)\) between \(x=a\) and \(x=b\) is: \[ Q=\int_a^b \delta_e(x)\,dx \]

Find the net charge on an \(8\,\text{m}\) bar whose linear charge density is \(\delta_e(x)=(1-x)\dfrac{\text{coul}}{\text{m}}\) if \(x\) is measured from one end.

The plot shows a bar from x equals 0 to 8, which is shaded in two
colors, showing it has positive charge close to
x equals 0, and negative charge close to x equals 8.

The net charge is \[\begin{aligned} Q&=\int_0^8 \delta_e(x)\,dx =\int_0^8 (1-x)\,dx \\ &=\left[x-\dfrac{x^2}{2}\right]_{x=0}^8 =8-\dfrac{64}{2}=-24\,\text{coul} \end{aligned}\]

Here's one for you:

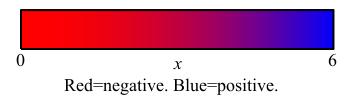
Find the net charge on an \(6\,\text{ft}\) bar whose linear charge density is \(\delta_e(x)=(x^2-4)\dfrac{\text{coul}}{\text{ft}}\) where \(x\) is measured from one end.

The plot shows a bar from x equals 0 to 6, which is shaded in two
colors, showing it has negative charge close to
x equals 0, and positive charge close to x equals 6.

\(Q=48\,\text{coul}\)

\[\begin{aligned} Q&=\int_0^6 \delta_e(x)\,dx =\int_0^6 (x^2-4)\,dx \\ &=\left[\dfrac{x^3}{3}-4x\right]_0^6 =\dfrac{216}{3}-24=48\,\text{coul} \end{aligned}\]

11. Mass and Center of Mass

d. Electric Charge

1. Bar with Non-Uniform Charge Density

Heading