18. Related Rates
b. Word Problems
There are many “real” world problems involving related rates. Here are some.
A cone which is \(H=16\,\text{cm}\) high and \(R=4\,\text{cm}\) in radius
is filled with water which is leaking out the bottom at
\(5\dfrac{\text{cm}^3}{\text{min}}\). How fast is the height of the water
decreasing when the water is \(10\,\text{cm}\) deep?
HINTS: Let \(h\) and \(r\) be the height and radius of the water in the
cone as functions of time. The volume of a cone is
\(V=\dfrac{1}{3}\pi r^2h\).
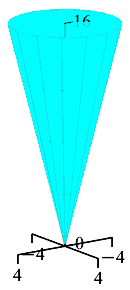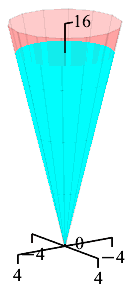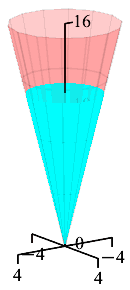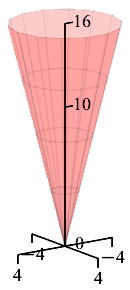
We start with the volume of a cone: \[ V=\dfrac{1}{3}\pi r^2h \] By similar triangles, the ratio between the height of the water and the radius of the water is: \[ \dfrac{r}{h}=\dfrac{R}{H}=\dfrac{4}{16}=\dfrac{1}{4} \] This means that we can substitute \(r=\dfrac{1}{4}h\) in the volume: \[ V=\dfrac{1}{48}\pi h^3 \] We take the derivative: \[ \dfrac{dV}{dt}=\dfrac{1}{16}\pi h^2\dfrac{dh}{dt} \] The current height is \(10\,\text{cm}\) and the volume is decreasing at \(\dfrac{dV}{dt}=-5\,\dfrac{\text{cm}^3}{\text{min}}\). We plug in and solve for the rate of change of the height: \[ -5=\dfrac{1}{16}\pi 10^2\dfrac{dh}{dt} \] \[ \dfrac{dh}{dt}=-\,\dfrac{80}{100\pi}=-\,\dfrac{.8}{\pi} \] So, the height is decreasing at a rate of \(\dfrac{dh}{dt}=-\,\dfrac{.8}{\pi}\,\dfrac{\text{cm}}{\text{min}}\).
When two resistors with resistances \(R_1\) and \(R_2\) are combined in parallel, the resulting resistance \(R\) satisfies: \[ \dfrac{1}{R}=\dfrac{1}{R_1}+\dfrac{1}{R_2} \] Currently, \(R_1=100\,\text{ohms}\) and is increasing at \(\dfrac{dR_1}{dt}=18\,\dfrac{\text{ohms}}{\text{hr}}\rule[-10pt]{0pt}{15pt}\), while \(R_2=200\,\text{ohms}\) and is decreasing at \(\dfrac{dR_2}{dt}=-9\,\dfrac{\text{ohms}}{\text{hr}}\rule[-10pt]{0pt}{15pt}\). Find the current values of \(R\) and \(\dfrac{dR}{dt}\rule[-10pt]{0pt}{15pt}\). Is \(R\) increasing or decreasing?
This time three variables are related.
\(R\) is increasing at \(\dfrac{dR}{dt}=7\,\dfrac{\text{ohms}}{\text{hr}}\)
This time three variables are related. We first find \(R\): \[\begin{aligned} \dfrac{1}{R}&=\dfrac{1}{R_1}+\dfrac{1}{R_2} =\dfrac{1}{100}+\dfrac{1}{200}=\dfrac{3}{200} \\ R&=\dfrac{200}{3} \end{aligned}\] Next, we differentiate the relation, using the Chain Rule: \[ -\,\dfrac{1}{R^2}\dfrac{dR}{dt} =-\,\dfrac{1}{(R_1)^2}\dfrac{dR_1}{dt}-\dfrac{1}{(R_2)^2}\dfrac{dR_2}{dt} \] We cancel the minuses, plug in numbers and solve for \(\dfrac{dR}{dt}\): \[\begin{aligned} \dfrac{9}{200^2}\dfrac{dR}{dt} &=\dfrac{1}{100^2}(18) +\dfrac{1}{200^2}(-9) \\ \dfrac{dR}{dt} &=\dfrac{200^2}{9}\left(\dfrac{1}{100^2}(18) -\dfrac{1}{200^2}(9)\right) \\ &=8-1=7\,\dfrac{\text{ohms}}{\text{hr}} \end{aligned}\] Since this is positive, \(R\) is increasing.
You should do lots of problems in the exercises.
Heading
Placeholder text: Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum