3. Trigonometry

d. Trig Identities

7. Summary of Trig Identities

Here is a summary of the identities which are most useful throughout calculus. * = more important   ** = most important

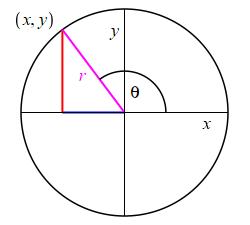
Circle Definitions

Quotient and Reciprocal Identities

\[\begin{aligned} &\tan\theta=\dfrac{\sin\theta}{\cos\theta}\quad &&\cot\theta=\dfrac{\cos\theta}{\sin\theta}=\dfrac{1}{\tan\theta} \\[8pt] &\sec\theta=\dfrac{1}{\cos\theta}\quad &&\csc\theta=\dfrac{1}{\sin\theta} \end{aligned}\]

Pythagorean Identities

\[\begin{aligned} &\sin^2\theta+\cos^2\theta=1 \qquad \qquad (**) \\ &\tan^2\theta+1=\sec^2\theta \\ &1+\cot^2\theta=\csc^2\theta \end{aligned}\]

Parity Identities

\[\begin{aligned} \sin(-\theta)=-\sin(\theta) \qquad \cos(-\theta)=\cos(\theta) \qquad \qquad \quad (*) \end{aligned}\]

Complementarity Identities

\[\begin{aligned} \sin\left(\dfrac{\pi}{2}-\theta\right)=\cos(\theta) \qquad \cos\left(\dfrac{\pi}{2}-\theta\right)=\sin(\theta) \qquad \quad (*) \end{aligned}\]

Supplementarity Identities

\[\begin{aligned} \sin(\pi-\theta)=\sin(\theta) \qquad \cos(\pi-\theta)=-\cos(\theta) \end{aligned}\]

Sum Identities

\[\begin{aligned} \sin(A+B)&=\sin(A)\cos(B)+\cos(A)\sin(B) \qquad \qquad (**) \\ \cos(A+B)&=\cos(A)\cos(B)-\sin(A)\sin(B) \qquad \qquad (**) \end{aligned}\]

Difference Identities

\[\begin{aligned} \sin(A-B)&=\sin(A)\cos(B)-\cos(A)\sin(B) \\ \cos(A-B)&=\cos(A)\cos(B)+\sin(A)\sin(B) \end{aligned}\]

Double Angle Identities

\[\begin{aligned} \sin(2A)&=2\sin(A)\cos(A) \qquad \qquad \quad \ (*) \\ \cos(2A)&=\cos^2(A)-\sin^2(A) \qquad \qquad (*) \\ &=2\cos^2(A)-1 \\ &=1-2\sin^2(A) \end{aligned}\]

Square Identities

\[\begin{aligned} \sin^2(A)&=\dfrac{1-\cos(2A)}{2} \qquad \qquad (**) \\ \cos^2(A)&=\dfrac{1+\cos(2A)}{2} \qquad \qquad (**) \end{aligned}\]

Law of Sines

Given a triangle with angles \(A\), \(B\) and \(C\) and opposite sides with lengths \(a\), \(b\) and \(c\): \[\begin{aligned} \dfrac{\sin A}{a}=\dfrac{\sin B}{b}=\dfrac{\sin C}{c} \end{aligned}\]

Law of Cosines

Given a triangle with angles \(A\), \(B\) and \(C\) and opposite sides with lengths \(a\), \(b\) and \(c\): \[\begin{aligned} c^2=a^2+b^2-2ab\cos C \qquad \qquad (**) \end{aligned}\]

The Triangle Inequality

Given a triangle with side lengths \(a\), \(b\) and \(c\): \[\begin{aligned} |a-b| \le c \le a+b \end{aligned}\]